如图所示,假设已知坐标系 ( X , Y ) (X,Y) (X,Y),旋转后的坐标系为 ( X ′ , Y ′ ) (X',Y') (X′,Y′),旋转角度为 θ \theta θ,假设点p在 ( X , Y ) (X,Y) (X,Y)坐标系下为 ( x , y ) (x,y) (x,y),坐标在旋转后的坐标系(即 ( X ′ , Y ′ ) (X',Y') (X′,Y′))中的坐标为 ( l , w ) (l,w) (l,w)。
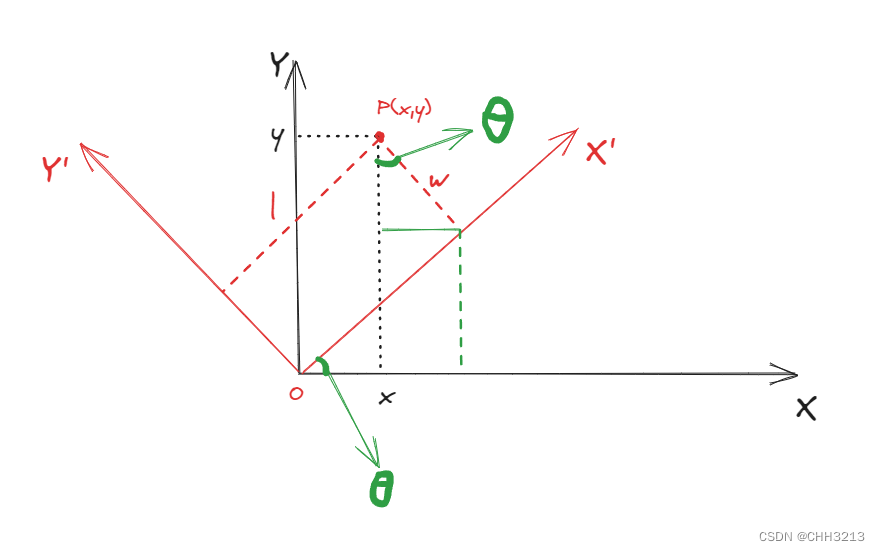
根据图中几何关系,可以表示出 ( x , y ) (x,y) (x,y)与 ( l , w ) (l,w) (l,w)的关系。
x = l cos θ − w sin θ y = l sin θ + w cos θ x=l\cos\theta-w\sin\theta\\ y=l\sin\theta+w\cos\theta x=lcosθ−wsinθy=lsinθ+wcosθ
即坐标转换公式为
[ x y ] = [ cos θ , − sin θ sin θ , cos θ ] [ l w ] \begin{bmatrix} x \\ y \end{bmatrix}=\begin{bmatrix} \cos\theta, \ -\sin\theta \\ \sin\theta, \ \cos\theta \end{bmatrix} \begin{bmatrix} l \\ w \end{bmatrix} [xy]=[cosθ, −sinθsinθ, cosθ][lw]
加上平移,如下图所示,假设 ( X ′ , Y ′ ) (X',Y') (X′,Y′)原点 O ′ O' O′在 ( X , Y ) (X,Y) (X,Y)坐标系下的坐标为 ( x 0 , y 0 ) (x_0,y_0) (x0,y0),则有以下关系式
[ x y ] = [ cos θ , − sin θ sin θ , cos θ ] [ l w ] + [ x 0 y 0 ] \begin{bmatrix} x \\ y \end{bmatrix} =\begin{bmatrix} \cos\theta, \ -\sin\theta \\ \sin\theta, \ \cos\theta \end{bmatrix} \begin{bmatrix} l \\ w \end{bmatrix}+\begin{bmatrix} x_0 \\ y_0 \end{bmatrix} [xy]=[cosθ, −sinθsinθ, cosθ][lw]+[x0y0]

[ cos θ , − sin θ sin θ , cos θ ] \begin{bmatrix} \cos\theta, \ -\sin\theta \\ \sin\theta, \ \cos\theta \end{bmatrix} [cosθ, −sinθsinθ, cosθ] 其实就是旋转矩阵。
分割线–未完待续