（说了好多话好多好多）POJ - 3070

转-知乎王希：https://www.zhihu.com/question/28062458

Description

In the Fibonacci integer sequence, F0 = 0, F1 = 1, and Fn = Fn − 1 + Fn − 2 for n ≥ 2. For example, the first ten terms of the Fibonacci sequence are:

0, 1, 1, 2, 3, 5, 8, 13, 21, 34, …

An alternative formula for the Fibonacci sequence is

.

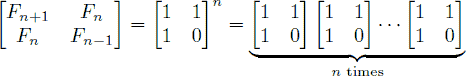Given an integer n, your goal is to compute the last 4 digits of Fn.

Input

The input test file will contain multiple test cases. Each test case consists of a single line containing n (where 0 ≤ n ≤ 1,000,000,000). The end-of-file is denoted by a single line containing the number −1.

Output

For each test case, print the last four digits of Fn. If the last four digits of Fn are all zeros, print ‘0’; otherwise, omit any leading zeros (i.e., print Fn mod 10000).

Sample Input

0
9
999999999
1000000000
-1

Sample Output

0
34
626
6875

Hint

As a reminder, matrix multiplication is associative, and the product of two 2 × 2 matrices is given by

.

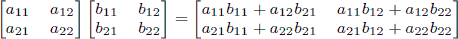Also, note that raising any 2 × 2 matrix to the 0th power gives the identity matrix:

.

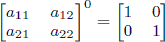Source

Stanford Local 2006

本题用到矩阵的快速幂：

首先，斐波那契可以由矩阵求得：

 由此推得

     

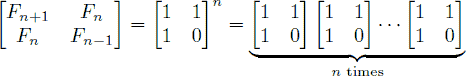
 初始矩阵为，记为E，那么E^2 为，E^3为，E^4为......可以发现，（0,1）位置的数是第i个斐波那契数。

问题转化为求初始矩阵的n次方，是几次方斐波那契数对应的就是就是矩阵的位置

更详细的快速幂讲解参考https://www.cnblogs.com/cmmdc/p/6936196.html

#include <iostream>
using namespace std;
const int MOD = 10000;///最后的模
struct matrix///定义矩阵结构体
{
    int m[2][2];
} ans, base;
matrix multi(matrix a, matrix b)///定义矩阵乘法。a,b代表两个矩阵
{
    matrix tmp;///求和矩阵
    for(int i = 0; i < 2; i++)
    {
        ///第一个矩阵的行
        for(int j = 0; j < 2; j++)
        {
            ///第二个矩阵的列
            tmp.m[i][j] = 0;///和初始化
            for(int k = 0; k < 2; k++)
                tmp.m[i][j] = (tmp.m[i][j] + a.m[i][k] * b.m[k][j]) % MOD;
        }///注意这时候要取一次模
    }
    return tmp;///返回结构体和的矩阵
}
int fast_mod(int n)///求基础矩阵base的n次幂
{
    base.m[0][0] = base.m[0][1] = base.m[1][0] = 1;
    base.m[1][1] = 0;///构造初始矩阵
    ans.m[0][0] = ans.m[1][1] = 1;///普通快速幂ans初始化为1
    ans.m[0][1] = ans.m[1][0] = 0;///那么矩阵ans就应该初始化为单位矩阵
    while(n)
    {
        if(n&1)  ///实现ans*=t;其中要先把ans赋值给tmp，然后用ans=tmp*t
            ans = multi(ans, base);
        base = multi(base, base);///全部转化为矩阵乘法
        n >>= 1;
    }
    return ans.m[0][1];///返回矩阵的（0,1）位置
}
int main()
{
    int n;
    while(cin>>n)
    {
        if(n==-1)
            return 0;
        cout<<fast_mod(n)<<endl;
    }
}