(1) Object: the foundation of all things - number and shape ( the two pillars of science and mathematics, the commonality and essence of all things, and the two sides of a thing, the two are closely related )

① Changes in shape

②Increase or decrease in volume

(2) Content: Mystery of all things, the principle of all things - research on commonality, essence, and law (mathematics is a science that studies patterns and orders)

Discover the essence, reveal the law, establish connections, seek commonalities

①Invariance: There is stillness in movement, constancy in change, order in chaos, similarity in difference, rationality in emotion, and usefulness in reason

② classification

Concept: classification of things → commonality

Conclusion: theorems, formulas, rules

2. Mathematical ideas and methods

1. The program established by mathematics

(1) Mathematics development stage

① Stages of the innovation process

②Theory building stage

③Application stage

2. The method of mathematical establishment

(1) Classification research: Classify by object attribute, research one by one

Difficult to easy, complex to simple, whole to zero, accumulation of zero to whole

(2) Reduction method

(3) Analogy method

(4) Induction method

(5) Abstraction

(6) Symbolization

(7) Axiomatic

(8) Optimization

(9) Modeling

3. Characteristics and Status of Mathematics

1. Starting point: the abstraction of the concept

(1) Abstract function: find commonality and grasp the essence

(2) Features of mathematical abstraction:

a. Focus only on numbers and shapes

b. Deepen layer by layer and improve step by step

c. It is almost completely involved in abstract concepts and their interrelationships

The formation of concepts or definitions in mathematics is mainly the result of classification, grasping the essence, and grasping the commonality

2. Process: rigor of reasoning

(1) Plausible reasoning:

Induction (individual → group; specific → general; innovative)

Analogy ( from general to specific, one individual knows another ) ...

(2) Deductive reasoning ( convergent thinking )

a. Starting from a few known facts, derive a rich body of knowledge

b. It can guarantee the correctness of mathematical propositions and make mathematics invincible

c. It can overcome the limitations of instruments, technologies and other means, and make up for the inadequacy of human experience

d. Make the scope of human cognition go from limited to unlimited

e. Provides an effective form for human beings to construct theories

3. Conclusion: the certainty of the conclusion

Deductive reasoning → Logically deterministic and reliable (uniqueness is not guaranteed)

4. Results: Extensive application

5. The status of mathematics: basic, universal, reliable

topic:

02 Mathematics

1. Functions of mathematics

1. Practical functions: the foundation of all things (universal and reliable, universal from objects, reliable from methods), life assistant (calculation, measurement), mother of science, servant of science

2. Educational functions: knowledge, tools, abilities (intelligence, comprehension, analysis and judgment, acumen, good at induction, insight into the essence, rational mathematical thinking), culture

3. Language functions: symbols, formulas, rules, theorems, equations...

Three characteristics: simplification, clarity and expansion

4. Cultural function

(1) Deepen human understanding of the world and promote the development of human material and spiritual civilization

(2) Long-term accumulation and precipitation, sublimation of human spirit and character

① It is the knowledge, method, and thought created and passed down by human beings

②Into every corner of society

③Influences human thought, promotes technological development and social progress, and is closely related to other cultures

Mathematical cultural connotation: intellectual component (mathematical knowledge), conceptual component (mathematical concept system)

2. The value of mathematics

1. Teaching and personal growth: scientific quality (mathematics), humanistic quality (literature), artistic quality (physical senses) → truth, goodness and beauty

(1) Mathematics awareness

(2) Mathematics language: symbolization and modeling

(3) Mathematics skills: methods and means

(4) Mathematical thinking:

Abstraction (discovering essence, commonality)

Creativity (divergent thinking)

Logical (deductive reasoning, precise and rigorous)

Modeling (essential commonality → building a model)

2. Mathematics and human life

(1) Improve efficiency Example: 20 liters of water to wash clothes

(2) Explain the question Example: A chair with four legs (equal length) must be able to be placed stably on an uneven floor

(3) Rational judgment

(4) Scientific decision-making

3. Development of Mathematics and Technology

(1) Mathematics is the language of science (models describe nature)

(2) Mathematics is the mother of science (thinking breeds science) → classical mechanics, relativity, singularity theory, life science, topology, quantitative genetics, epidemiology...

(3) Mathematics is the maid of science (tools serve science) → Geological exploration, pollution issues

4. Mathematics and social progress

(1) Mathematical tools are an important force to promote material civilization (such as the Industrial Revolution)

(2) Mathematical rationality is an important factor in promoting spiritual civilization (such as axiomatic thinking)

(3) Mathematical aesthetics is a cultural hormone that promotes the development of art

03 Math Journey

1. Classification of Mathematics

1. Vertical—History

(1) Elementary mathematics and ancient mathematics (before the 16th century):

Euclidean geometry established in ancient Greece;

Arithmetic established in ancient China, ancient India, and ancient Babylonia;

Algebraic equations developed during the European Renaissance in the 13th and 15th centuries, etc.

Features: constant math

(2) Variable mathematics (17th-early 19th century)

① In the 17th century, French Descartes established analytical geometry (starting point)

② Newton and Leibniz established calculus (sign)

Features: combination of numbers and shapes, from static to motion, from logic to algebra

(3) Modern Mathematics (19th Century): The Second Mathematical Crisis

Analytical Rigor : Limit Theory

Algebraic abstraction : the existence, number, and structure of solutions → Abel: The general root-finding formula for quintic algebraic equations does not exist → Galois Group Theory

geometric de-Europeanization

(4) Modern mathematics (20th century)

Starting point: Hilbert proposed 23 mathematical problems in 1900

Features: more branches, cross enhancement

Basics: Cantor Set Theory

Features: Univariate to multivariable, low-dimensional to high-dimensional; from linear to nonlinear; local to global, simple to complex; continuous to discontinuous, stable to bifurcated; precise to fuzzy; computer applications

Trends: staggered development, highly comprehensive, and gradually unified; marginal, comprehensive, and interdisciplinary disciplines are increasing day by day; mathematical expressions, objects, and methods are increasingly abstract

2. Horizontal—objects and methods

(1) Objects and methods

Basic Mathematics: Algebra, Geometry, Analysis

Applied Mathematics: the application of research mathematics, mathematics that can be applied

Computational Mathematics: research calculation methods, involving approximate calculation and optimization

Probability and Statistics: Studying the Science of Randomness

Operations Research and Cybernetics: Management

(2) Natural phenomena: definite phenomena, random phenomena, fuzzy phenomena, extension phenomena

(3) Mathematical function angle:

Metric → Geometry (Topology)

Computing → Algebra (Algebraic Structures)

Statistics→Statistics

Compare → Analyze

2. Overview of the development of branches of mathematics

1. General Theory of Geometry: Epistemology of the Essence of Space

Research objects: "geometric objects", the geometric quantity of graphics, and the abstraction of spatial forms

Research content: the relationship and mutual position of various geometric quantities

Research methods: experimental method→speculative method→analytical method→vector method (vector geometry: analytic geometry that does not depend on the coordinate system)→calculus method...

2. Grand View of Algebra

Elementary algebra: study real numbers, complex numbers, the central problem → the existence, number and structure of solutions

Advanced Algebra:

Linear algebra: The unknown quantity of the equation increases, and the order remains the same → matrix, determinant, vector...

Polynomial algebra: the number of unknowns is small, but the degree is large → equation theory

Any polynomial equation has solutions in the complex range

3. The meaning of calculus:

Research object: function

Research Tools: Limits

Research content: function integration, function differentiation, fundamental theorem of calculus (a bridge linking differentiation and integration)

4. Stochastic mathematics and fuzzy mathematics

Stochastic Mathematics: Probability Theory and Mathematical Statistics

Fuzzy Math: Fuzzy Sets

3. Factors in the Formation and Development of Mathematics

1. Practical: the first driving force, social needs Geometry, calculus, probability theory

2. Science: rational structure of natural phenomena Graph theory, topology, complex variable functions

3. Philosophy: Intellectual curiosity, strong interest in pure thinking, number theory, non-Euclidean geometry, combinatorics

4. Aesthetics: the pursuit of beauty

Concrete → Abstract → Concrete

conjecture → proof

04 Theory of Mathematics

1. Mathematical thinking and its value

1. Classification

(1) Divergent thinking ( reasonable reasoning ): based on sensibility, feelings, feelings → conclusions may not be reliable

Induction (individuals know groups; from specific to general, innovative)

Analogy (one individual knows another individual)

Association, radiation, migration, spatial imagination, association , etc.

(2) Convergent thinking ( deductive reasoning ): general to specific → inevitability, correctness

Deductive reasoning such as syllogism, finite exhaustive method, mathematical induction method, and method of contradictory (indirect proof method) can derive a rich knowledge system from a few known facts.

Deductive reasoning can ensure the correctness of mathematical propositions and make mathematics invincible. Deductive
reasoning can make the scope of human cognition from limited to infinite

2. Mathematical Thinking in Mathematical Stories

1. What does mathematics focus on?

(1) Plato's Academy: "Those who do not understand geometry are not allowed to enter" → exercise thinking and enlighten wisdom

(2) Tell them where they are: Physicists, engineers → completely correct, no answers at all → pursue the truth

(3) Description of two sheep: 1+1=2→abstract generalization→grasp essence, commonality and law

(4) The sum of the interior angles of a triangle: Shiing-shen Chern → the sum of the exterior angles of an N-gon 360° → emphasizing invariance and invariance

2. How to think in mathematics

(5) Overthrow Fermat's Last Theorem (Andrew Wells 1994): x^n + y^n = z^n

Fermat's Last Theorem: When the exponent n is greater than 2, there is no integer solution.

→ 100 positive examples cannot confirm the truth, but one negative example can overthrow the truth

(6) The story of boiling water: return to thought

(7) How tall is the Diwang Building: benchmark projection method, mirror reflection method → analogy thinking

(8) Baldy World: Induction and Deduction

(9) Fence area: engineers, physicists, mathematicians → reverse thinking

(10) Color of hats: two black hats, three white hats → hypothesis exclusion, proof by contradiction

3. How to express mathematics

(11) Scottish sheep: fine and rigorous

(12) There are a few birds in the tree: really dead, silent pistol, deaf → combination of divergence and rigor

3. Mathematical thinking in mathematical games

1. Hide Thirty:

(1) How to play 1: The two sides take turns to count, count at least one number in each round , and at most three numbers , and the person who counts to 30 at the end loses

→ Multiples of 4 plus one

(2) How to play 2: The two sides take turns to report the number. Each round counts at least one number and at most two numbers . The person who counts to 30 at the end loses. How can the first player guarantee to win?

(3) How to play 3: The two sides take turns to count. Each round counts at least two numbers and at most four numbers . The person who counts to 40 at the end loses. Is there a trick to win first?

2. Taking stones: several piles of stones, the two sides take turns to take stones, only 1-3 stones can be taken each time, and the winner is to get the last stone

→ specialization

(1) A pile: leave stones in multiples of 4 (if 30 → take two stones first)

(2) Two piles: the remaining pile is a multiple of 4 → the remainder is the same when divided by four

(3) Arbitrary heap: reduction

3. Deformation to take stones: several piles of stones, both sides take turns to take stones, each time you can take any stone, and the last stone is the winner

(1) A pile: take it all at once

(2) Two piles: ensure that the number of stones in the two piles is the same

(3) Three stacks:

a. There are at least two piles of the same number of stones: the first player takes out the remaining piles of stones with different numbers, and leaves two piles of the same stones for the opponent.

b. The three piles of stones are different from each other (m>n>k): the situation where 1, 2, and 3 stones are left to the opponent can seal the victory

→ Leave it to the opponent (1, 2m, 2m+1) to win the game.

4. The characteristics of taking stones to win the game

Binary → even endgame must be a winning game (the digits of the addition result are all even numbers, no carry processing)

reason:

a. The even-type endgame must become an odd-type endgame after taking a child;

b. For any odd-shaped endgame, there must be a way to make it become an even-shaped endgame after taking a child.

05 Mathematical Debate

1. There is stillness in the movement

1. The Euler line of a triangle: orthocenter, inner center, circumcenter

2. Symmetry: axis, center rotation

3. Fixed point theorem

Two, there is constant in change

1. Changing constants

2. Relationships in change: the sum of geometric sequence fragments, Fibonacci sequence

3. Identity in change: identity transformation, same-solution transformation, elementary transformation → one form is reduced to another form

3. Order in chaos

1. Any set of numbers has certain characteristics (essence) in a certain sense

2. Any several groups of numbers are related to some extent (regularity)

3. Statistical laws of random phenomena

Fourth, there are similarities in differences

5. Be reasonable

1. Honeycomb construction: dense paving, material volume, maximum area → regular hexagon

2. Drawer principle: birthdays (more than 23 people, more than 50% have birthdays on the same day), in a party, there must be two people present with the same number of friends

6. Reasonable and useful

1. Sources of mathematics: practical needs, mathematical development, and human curiosity

2. Various averages: arithmetic mean→distribution, geometric mean→geometric→balance weighing, harmonic mean→music

3. Euler's theorem → RSA coding → it is easy to multiply two large prime numbers, but it is difficult to decompose a large integer

4. Evidence by contradiction

5. The principle of square and radius, the way of being a man: 80/20 rule

6. Total probability formula (Bayesian formula)

06 The beauty of mathematics

1. The source and characteristics of beauty

Beauty is a characteristic that should be met objectively and satisfied with subjective feeling and experience → aesthetic object, aesthetic subject → natural attribute, social attribute

1. The perceptual characteristics of beauty (external): simplicity (simple solutions to complex problems), harmony (symmetry, order, regularity), singularity

2. Rational features of beauty (internal): average face experiment → social and common

3. The root of mathematical beauty

(1) Research object and content: Mathematics focuses on the essence, commonality, law, and connection, and has the beauty of simplicity and harmony

(2) Research methods and ways of thinking: Mathematics reflects nature and society, attaches great importance to changes and special cases, and has a unique beauty

4. How to appreciate the beauty of mathematics: use thinking to feel and think

5. The beauty of simplicity in mathematics: focus on the essence and commonality → the simplicity of proof methods, expressions, and theoretical architecture

(1) The beauty of symbols: a sentence → a symbol Example: factorial, power

(2) The beauty of constants: constant in change, static in dynamic

Five important constants: 0 1 i e pi (i-imaginary unit)

→ Euler's formula: f-e+v=2 (number of faces - number of edges + number of vertices = 2)

(3) The beauty of unity: the connection of different things Example: Gaussian unified Euclidean geometry, Riemannian geometry, Lobachevsky geometry

6. The beauty of harmony in mathematics: whole and part, part and part

(1) The beauty of symmetry: the invariance of all things in change → symmetrical, balanced, similar, stable

(2) The beauty of sequence: the order, correlation, and regularity of everything in change

(3) Rhythm beauty

7. The strange beauty of mathematics

(1) Limited beauty: limited knowledge expresses the infinite

(2) Mysterious beauty: unbelievable but correct

(3) Contrasting Beauty: Huge Contrast of Mutation Phenomena

(4) Funny beauty: Abnormal mathematical thinking and funny phenomena in real life

2. The beauty of mathematical methods

1. A leap in epistemology: understanding the infinite with the finite

Mathematical induction: a bridge between infinity and finiteness, a reasoning technique to establish propositions related to natural numbers

Origin, transfer relationship

Proof by contradiction: prove the falsity of a negative conclusion to confirm the authenticity of a positive conclusion

infinite/complex → finite/simple

2. The beauty of deductive method: demonstrating complexity with simplicity

Reasoning from the general to the particular, first there is a general law, and then the nature of a specific case is derived from the law

Syllogism: major premise, minor premise (a judgment about a particular object), conclusion

Guarantee of Invincibility in Mathematics

3. The beauty of analogy

n-degree polynomial → Veda's theorem → the relationship between the root of Euler's sine function and Taylor's coefficient

4. The beauty of the combination of numbers and shapes

Pythagorean Theorem: In and Out Complementary Graphs

anti-correction formula

5. Here the invisible is better than the visible

Existence problem proof: constructive proof (concretely construct the object), pure rational proof (deduce the existence of the object theoretically)

Drawer principle, law of excluded middle (example: there are two irrational numbers a and b, making a^b a rational number)

6. From low-level mathematics to high-level mathematics: solve problems that cannot be solved by low-level mathematics, and gain a deeper understanding

3. The beauty of mathematical conclusions

1. The beauty of triangles

(1) Stability

(2) Five centers of a triangle: center of gravity (central line), circumcenter (perpendicular line), inward center (angle bisector), orthocenter (perpendicular line), side center (one angle bisector and the other two exterior angle bisectors)

2. The beauty of a circle: pi

(1) Zu Chong's secret rate (355/113): accurate, simple, easy to remember (113355), wonderful

(2) Bronkel, Leibniz, Newton, Euler...

Can be expressed through all natural numbers and all odd numbers

3. The beauty of rectangle

(1) Aspect ratio √2 format

(2) Golden section number: 0.618

4. The beauty of the Fibonacci sequence

(1) Digital guessing: a(n+1)=an*1.618

(2) Fibonacci sequence: 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233...

Natural phenomena: conch, sunflower spiral

Example: climbing stairs

Essence: the law of inheritance, its offspring is determined by the previous two generations

(3) Nature

①Sum: Starting from the third item, each item is the sum of the previous two items

② difference: an+2 - an+1 = an

③ Product: The product of any two adjacent items is equal to the sum of the squares of the smaller item and its items

④ product: the product of any two terms is equal to the square of the middle number ±1

an-1*an+1=an^2+(-1)^n

⑤The product of any two terms with an odd number of terms is equal to the square of the middle number ±1

⑥ Quotient: The quotient of any adjacent two terms tends to a stable value of 0.618 → the quotient of any adjacent preceding two terms tends to a stable value of 1.618

⑦ Remainder law: the remainder of each item divided by a certain number is periodic, and each finite item has a cycle (including any sequence generated in a recursive form)

⑧The sum of the first n items: a1+a2+......+an = an+2 - 1 = an+2 - a2

⑨The sum of any adjacent ten items: the second number after the ten items minus the second number in the ten items

5. The base of natural logarithms

(1) Maximum compound interest problem

Suppose the annual interest rate of demand deposit is 100% → 1 yuan → 2 yuan

Withdraw the deposit in half a year and deposit it in the bank → 1 yuan → 1.5 yuan → 1.5+1.5*50%=2.25 yuan

Withdraw on a quarterly basis and then deposit → 1.25^4≈2.44 yuan

→If the interest is divided into n installments a year, the interest rate for each installment is 1/n, the deposit is 1 yuan, and the sum of principal and interest at the end of the year is (1+1/n)*n yuan

→ Constantly increases with n, approaching an infinite transcendental number → e≈2.72

(2) Exponential function

(3) Distribution law of prime numbers

(4) Complex numbers on the unit circle: Euler's formula

(5) Normal distribution curve

07 Mathematical Wonders

1. Real number system

1. Overview of the expansion of the number system: "Number" is one of the two cornerstones of mathematics. The emergence and development of various numbers are derived from practical needs or the development needs of mathematics itself.

(1) Natural number N: quantity, scale, order

(2) Integer Z

(3) Score (rational number Q): assign

(4) Negative number: in debt

(5) Irrational numbers: infinite non-recurring decimals, admitted in the 4th century BC (a milestone in the history of mathematics development) → observation, measurement, experiment, calculation, and experience are unreliable, and reasoning is reliable

(6) Real numbers R: positive numbers, negative numbers, rational numbers, irrational numbers → practical meaning, a continuous system without gaps

(7) Complex number C: The complex number system is the largest number system that maintains the basic properties of the four arithmetic operations

Appeared: In the late 15th century, in 1484, the French mathematician Shu Kai

Cardano: 1545 → Negative numbers can be square root

Descartes: 1637 → imaginary numbers imaginary numbers

Euler: 1777 → Introduction of i as imaginary unit

Gauss: 1799 → Geometric representation of complex numbers

1831 → Complex numbers denote points on a plane (leap) → Plane vectors → Calculations

→The plural is the soul of the plane

(8) Quaternion: Ordered quaternion real arrays can form a number system, multiplication is not commutative → quaternion field

(9) Octonions (super complex numbers): multiplication is not commutative, nor can it be combined

(10) Algebraic numbers: among real numbers, the roots of algebraic polynomials with integer coefficients , among which rational numbers are the roots of polynomials with integer coefficients of degree one ( all rational numbers are algebraic numbers )

(11) Transcendental numbers: Real numbers that are not algebraic numbers , such as pi and e. ( Irrational numbers may be algebraic numbers or transcendental numbers )

Inverse operation: natural number → negative number → integer; integer → division → fraction → rational number; natural number → square root → irrational number → real number; negative number → square root → imaginary number

2. Rational number set (the smallest number field): finite decimals, infinite recurring decimals

(1) Algebra: closed, forming a number field (square extraction operation, not closed for limit operation)

Number field: Four arithmetic operations are closed, addition and multiplication satisfy associative law and commutative law, multiplication and addition satisfy distributive law

(2) Geometry: dense on the number axis (still has gaps)

(3) Collection:

If a one-to-one correspondence can be established between the elements of sets A and B, it is considered that there are as many elements in A and B

If a one-to-one correspondence can be established between the elements of a certain subset of A and B, then the elements of A are considered to be no more than the elements of B

① How much: the set of rational numbers is countable

There are as many positive integers as there are positive even numbers, and there are as many positive integers as there are squares

Infinite sets like natural numbers that can be arranged in a row or counted one by one are called countable sets

② Rational number length is 0

3. Set of real numbers: rational numbers, irrational numbers (infinite non-recurring decimals)

(1) Algebra: closed

(2) Geometry: continuous and seamless on the number axis (closed limit calculation → calculus can be established)

(3) Geometry: uncountable sets → cannot establish one-to-one correspondence with natural numbers

4. The cardinality א of infinite sets (hereinafter, א is written as N)

Cardinality: The amount that describes the number of elements contained in a set A is called the cardinality of this set

(1) Operational properties of countable set base N0:

N0+n=N0

N0+N0=N0，nN0=N0

N0*N0=N0，（N0）^n=N0

(2) Algebraic number sets are countable sets → transcendental numbers are uncountable

(3) The set of real numbers is uncountable, and the base is N1 (continuum base) → irrational numbers are uncountable

Operation nature: N1+n+N0=N1

N1+N1=N1，nN1=N1

N0*N1=N1，N1^n=N1

(4) Transcendental numbers: Liouville number, e, π, speed of light, gravitational constant...

5. Recognize finite numbers (N0 and N1)

(1) The base of the power set: the power set of the set M → the set P(M) composed of all subsets of the set M

(2) Cantor's theorem (no maximum cardinality)

The set of real numbers is the power set of the set of rational numbers → N1=2^N0

N0: the number of all integers and rational numbers

N1: the number of all geometric points on lines, surfaces, and solids N1=2^N0

N2: the number of all geometric curves N2=2^N1

6. The continuum hypothesis: Are there any other bases between N0 and N1?

→ no

Gödel, Cohen: Yes or no

2. Coexistence of Three Geometries

1. Euclidean geometry

(1) Thales: The first person in the history of mathematics to provide geometric proofs

(2) Pythagoras: Pythagorean Theorem

(3) Euclid: screening theorems, selection axioms, rationally arranging content, carefully organizing methods → establishing mathematical axiomatic thinking

① Five general axioms

(a,b,c,d are all positive numbers)

a. Two quantities that are equal to the same quantity are equal; that is, if a=c and b=c, then a = b (equal quantity substitution axiom).

b. Equal amount plus equal amount, the sum is equal; that is, if a=b and c=d, then a+c = b+d (equal amount addition axiom).

c. The difference between the equal amount and the equal amount is equal; that is, if a=b and c=d, then ac = bd (equal amount subtraction axiom).

d. Two figures that are completely superimposed are congruent (axiom of transposition and superposition).

e. The total amount is greater than the component, that is, a+b>a (the total amount is greater than the component axiom).

② five geometric axioms

a. Through two different points, one can only draw a straight line (straight line axiom).

b. Line segments (finite straight lines) can be extended arbitrarily.

c. With any point as the center and any length as the radius, a circle can be constructed (circle axiom).

d. All right angles are equal (angle axiom).

e. Two straight lines are cut by a third straight line. If the sum of the two interior angles on the same side is less than two right angles, the two straight lines will intersect on that side.

2. Non-Euclidean geometry (Lobachevsky geometry + Riemannian geometry): Overturning the fifth postulate

(1) Gauss: In 1792, he began to think about the fifth postulate problem (Bowyer)

(2) Lobachevsky: " Through a point outside a straight line, two straight lines can be drawn that do not intersect with it " instead of the fifth axiom (birth date of non-Euclidean geometry: February 11, 1826)

(3) Riemann: including Lobachevsky geometry (curvature is a negative constant) and Euclidean geometry (curvature is always equal to 0), special Riemannian geometry (curvature is a positive constant)

3. Three geometric comparisons

(1) Euclidean geometry: daily small range, plane, triangle interior angle and 180°

(2) Lobachevsky geometry: roaming in space or nuclear world, three models (Poinsley, pseudo-sphere, Poincaré, Klein), the sum of the interior angles of a triangle is less than 180°, and the triangle area is large

(3) Riemannian geometry: long-distance travel on the earth, sphere, triangle interior angle sum is greater than 180°, triangle area is small

3. Hetu Luoshu and Magic Square

1. Know the magic square

(1) Luoshu: The sum of the three numbers on any horizontal, vertical and diagonal lines is equal to 15

(2) Magic square: a method of arranging numbers in a square grid so that the sum of numbers on each row, column, and diagonal is equal

①Standard magic square: If each number of the magic square is a continuous natural number from 1 to n^2, it is called a standard magic square

The magic sum of the standard magic square of order n is: n*(n^2+1)/2

(3) Why study the magic square: the beauty of magic, the power of enlightenment, and the need for application

(4) Classification:

① order

a. Odd order magic square

b. Even-order magic squares: double-even-order magic squares, single-even-order magic squares

②Properties: square magic square (double magic square) - the sum of the squares is also equal, and the product magic square (product magic square) - the multiplication of each number is also equal

(3) Existence: the second-order magic square does not exist, there is only one type of third-order magic square (Luoshu), and there are 880 kinds of fourth-order magic squares...

2. Construct a magic square

(1) Odd order - Yang Hui:

The structure of the third-order magic square (odd-numbered order): Nine sons are arranged diagonally, up and down are reversed, left and right are changed, and four-dimensional advancement

Result: wear nine shoes, one on the left, three on the right, seven on the right, two and four on the shoulders, six and eight on the feet

(2) Odd order-Rauber construction:

In a square matrix with (2n+1)*(2n+1) squares, fill in the middle of the top row with 1, and then proceed according to the following rules:

One is in the center of the top row, and the last numbers are placed on the upper right in turn.

When the top is out of the way, put it on the bottom, and when the right is out of the way, lie on the left.

The weight row is moved from below, and the right top flies to the left bottom.

(3) Even order-Haier structure:

Root number: nth order magic square → the root number of p is n(p-1)

Diagonal row: 1→n fills in two diagonal lines from left to right (square matrix A)

Reverse order filling: Fill in the remaining numbers that are not in 1→n in each row (square matrix B)

Square matrix transposition: square matrix B transpose (exchange rows and columns) to get square matrix C (original number)

Root number replacement: original number → root number → square matrix D

Square matrix merge: square matrix B and D merge

(4) Bieven-order magic square

Complement: nth order magic square→p's complement is n^2+1-p

Fill in sequentially: 1→n^2

Diagonal line replacement: draw a diagonal line every two times + the original diagonal line → replace the complement

3. Appreciate the magic square

08 Math fun

1. Fun with numbers

Digital black hole: digital string → after repeated calculations with certain rules, the same result can be obtained

1. 6174 black hole (Capreca black hole):

(1) Randomly choose a four-digit number (numbers cannot be all the same)

(2) Arrange from large to small → number 1, arrange from small to large → number 2

(3) Subtract the latter from the former to get a new number, repeat the above operation (Capreca transformation)

(4) Within 7 cycles, 6174 will be obtained

*Three-digit version (495, up to six steps)

*Two-digit version (multiples of 9)

2. 123 black holes (Sisyphus string):

(1) Take any number and count its even number, odd number and total number of digits

(2) Arrange according to (even, odd, total) to get a new number

(3) Repeat the above process, and finally a result of 123 will be obtained

*Why: There are only five cases for four digits → 404, 314, 224, 134, 044

→303、123、303、123、303→123

3. 153 black hole (narcissistic digital black hole): Take any multiple of 3, find the cube sum of its one-digit number as a new number, repeat the above process for the new number, and finally get the result of 153. (370, 371 are also similar black holes)

4. 1 and 4 black holes: for any natural number, find the sum of the squares of its digits as a new number, repeat the above process, and the final result must be 1 (black hole) or 4 (vortex)

5. 3x+1 problem: For any natural number, if it is an even number, divide it by 2; if it is an odd number, multiply it by 3+1, repeat the above operation, and the final result must be 1

2. The fun of numbers and shapes

1. Pythagorean Theorem——Geometric Viewpoint

Two treasures of geometry: Pythagorean Theorem (Pythagoras Theorem), Golden Section

2. Pythagorean Theorem——Algebraic Viewpoint

(1) In a right triangle whose side length is an integer: there must be a number in the Pythagorean that is a multiple of 3, there must be a number in the Pythagorean that is a multiple of 4, and there must be a number in the Pythagorean string that is a multiple of 5

(2) There is no combination in which Pythagorean is odd and string is even

(3) The sum and difference of a certain number in chord and Pythagorean are both perfect square numbers

(4) The arithmetic mean of a certain number in string and Pythagorean is a perfect square number

3. The Pythagorean Theorem—An Interesting Talk about Pythagorean Numbers

(1) In addition to 1 and 2, any natural number can be used as the length of a right-angled side of a right-angled triangle with an integer side length

(2) A right-angled side and hypotenuse are consecutive integers

(3) Two right-angled sides are consecutive integers

3. The fun of logic

1. Origin and definition of paradox

(1) Zeno's paradox:

① Motion does not exist: space and time are continuous and infinitely divisible, motion does not exist

②Achilles Paradox

③Flying arrows do not move (intermittent space-time concept)

④ Motion relativity

(2) Lying Paradox

2. Paradox and mathematical development

The First Mathematical Crisis (Ancient Greek Period): Recognition of Irrational Numbers → Eudoxus' New Proportional Theory

Experimental Mathematics → Inferential Mathematics → The Birth of Axiomatic Geometry and Logic

The Second Mathematical Crisis (18th Century): Instantaneous Velocity Problems, Curve Tangents, Function Extremum, Quadrature Problems → Newton, Leibniz Calculus → 19th Century Limit Theory

Changes in methodology → reasonable reasoning to find direction, deductive reasoning to draw conclusions

The third mathematical crisis: Cantor’s set theory → Russell’s paradox → axiomatization of set theory → ZFC system → Gödel’s incompleteness theorem

epistemological slack

3. Paradox example and enlightenment

paradox is inevitable

paradox can be resolved

The conditional convergent series does not satisfy the commutative law

3. Mathematics, games and magic

1. Existence and non-existence—binary magic: right to left → 1/0

2. Odd and even - which card was moved

3. Sequence and number - I know the secret of you two

(1) Pigeon cage principle: There must be two cards of the same suit among the five cards → take out one card and put the other card first

(2) There are three left → 1 ~ 6

09 Mathematics

1. The principle of mathematical induction

Laplace: In mathematics, our main tools for discovering truth are induction and analogy.

1. Theoretical basis:

Peano's axiom of natural numbers: The set of natural numbers N is a set that satisfies the following conditions

(1) There is an element recorded as 1

(2) Every element in N can find an element in N as its successor n+, 1 is not the successor of any element

(3) Different elements have different successors

(4) Inductive axiom : For any subset M of N, if 1∈M, and as long as n is in M, it can be deduced that n’s successor n+ is also in M, then M=N

2. Application

2. Evidence by contradiction and drawer principle

3. The Seven Bridges Problem and the One-Stroke Theorem

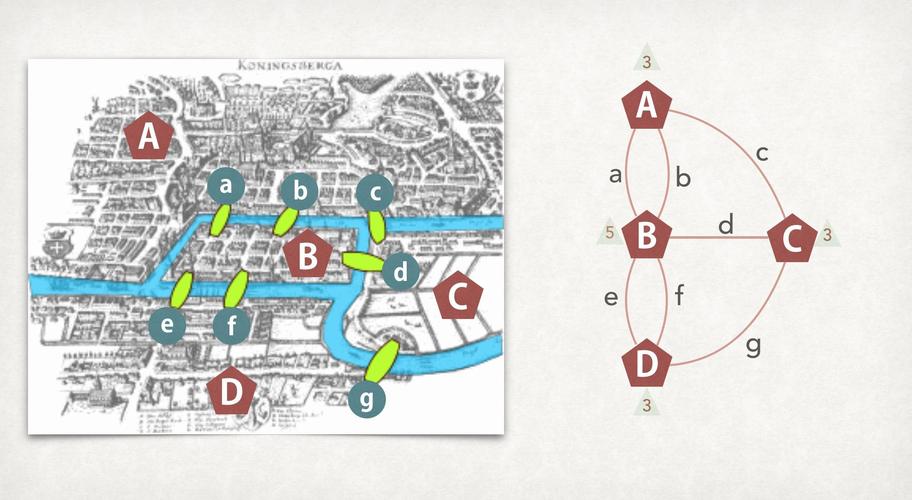
The number of singularities is at most 0 or 2 → the seven-bridge problem is impossible

4. Number theory and ciphers

1. Basic encryption mode: content (plaintext) → key → ciphertext

2. Substitution method: cracking → intercepting a large amount of information

3. RSA encoding method: the difficulty of factorizing extremely large integers determines the reliability of the RSA algorithm

（1）p、q→N=p*q

(2) The smaller number n makes n and (p-1) and (q-1) mutually prime

(3) m→mn-1 is a multiple of (p-1)(q-1)→mn = k(p-1)(q-1)+1

Publicly available: N, n

Encrypted transmission process (Euler's theorem):

(1) The remainder obtained from plaintext x → x to the nth power/N → ciphertext y

(2) The remainder obtained from ciphertext y → y to the m power/N → plaintext x

Example: p = 3 q = 11 p - 1 = 2 q - 1 = 10

A. N = 33, choose n = 7 → public key

B、mn - 1 = 7m - 1

= k*2*10 = 20k

→m = (20k + 1) / 7

C. Take k = 1, then m = 3

10 math questions

1. Three major mathematical problems in ancient times ( the use of algebraic methods in the 19th century proved all impossible )

Limitation: non-scale ruler, compasses, limited number of steps

1. Turn the circle into a square

(1) In the 5th century BC, the ancient Greek philosopher Anaxagoras was thrown into prison for "blasphemy" because he discovered that the sun was a big fireball, not the god Apollo.

(2) During the days waiting for execution, Anaxagoras could not sleep, and he became interested in the prison bars and the full moon. He kept changing the observation position, seeing the circle bigger than the square for a while, and seeing the square bigger than the circle for a while. Finally he said: "Okay, let's say the two figures have the same area."
(3) Anaxagoras took "construct a square so that its area is equal to the known area of a circle" as a ruler and compasses. graph problem to study. At first he thought that this problem was easy to solve, but unexpectedly he spent all his time and got nothing.
(4) After many rescues by his good friend and politician Pericles, Anaxagoras was released from prison. He published the problem he thought of in prison. Many mathematicians were very interested in this problem and wanted to solve it, but none of them succeeded. This is the famous "square the circle" problem

2, times the cube

(1) Legend has it that in the 4th century BC, a disease was prevalent in Athens in ancient Greece. In order to eliminate the disaster, the Athenians asked the sun god for help.

(2) The sun god said: "If you want to prevent the epidemic, you must double the volume of the cube incense table in front of my temple." This condition made the Athenians very happy. They thought it was easy to do, so they put the old Each edge of the incense case is doubled, and a new cube incense case is made. However, the epidemic has become more rampant

(3) When the Athenians went to pray to the sun god again, they realized that the volume of the new incense case was not twice that of the old incense case. This stumped people at that time, and even Plato, the most famous scholar, felt powerless.

3. Trisection angle

(1) In the 4th century BC, there was a circular villa on the outskirts of Alexandria, in which lived a princess. There is a river in the middle of the circular villa, and the princess's bedroom is built exactly at the center of the circle. A gate was opened on the north and south walls of the villa, and a bridge was built over the river. The position of the bridge and the north and south gates were exactly in a straight line. The items rewarded by the king every day were brought in from the north gate and put into the warehouse at the south gate first, and then the princess sent someone to retrieve the living room from the south gate.
(2) One day, the princess asked the attendant: "Which is the longer distance from the north gate to my bedroom, or from the north gate to the bridge?"
(3) After a few years, the princess's younger sister, the little princess, grew up, and the king wanted to build a villa for her. The little princess proposed that her villa should be built like her sister's villa, with a river, a bridge, and north and south gates. The king fully agreed, and the construction of the little princess' villa will start soon. When the south gate is built and the positions of the bridge and the north gate are determined, a problem arises: how can the north gate reach the bedroom and the north gate reach the bridge? How about the same distance?

4. Why the three major problems cannot be solved

(1) Turn the circle into a square

(2) times the cube

(3) Trisection angle

2. Fermat's conjecture ( solved )

Fermat's conjecture: When the integer n>2, there is no positive integer solution for x^n+y^n=z^n. (around 1637)

Euler proved that when n=3, the Fermat conjecture is true, published in "Guide to Algebra", the method is "infinite descent method"

3. Goldbach's conjecture ( unresolved, related to prime numbers )

Goldbach's conjecture: Any integer greater than 5 can be written as the sum of three prime numbers

Commonly used saying: Any even number greater than 2 can be written as the sum of two prime numbers

The proposition "any sufficiently large even number can be expressed as the sum of a number whose prime factors do not exceed a and another number whose prime factors do not exceed b" is written as "a+b".

In 1966, Chen Jingrun proved that "1+2" was established, that is, "any sufficiently large even number can be expressed as the sum of two prime numbers, or the sum of a prime number and a semi-prime number".

Four, four-color conjecture

has been solved by computer

Three modern mathematical problems: Fermat's conjecture, Goldbach's conjecture, four-color conjecture

5. Poincaré conjecture (proved by Perelman in 2006, the only one solved among the seven major problems)

1. Seven Millennium Problems: Baidu Encyclopedia - Verification

2. Poincaré conjecture content:

(1) Two-dimensional plane: A closed curve (including an infinitely long straight line and a point at infinity), no matter how complicated it is, is equivalent to a circle in a certain sense.

(2) Surface: A closed surface without holes, including infinite planes and infinite points, no matter how complex it is, is equivalent to a sphere in a certain sense.

(3) In 1904, French mathematician Henri Poincaré proposed a topological conjecture:

"Any simply connected, closed three-dimensional manifold must be homeomorphic to a three-dimensional sphere."

Simply put, a closed three-dimensional manifold is a three-dimensional space with boundaries; simple connectivity means that every closed curve in this space can be continuously shrunk to a point, or in a closed three-dimensional space, if each closed All curves can be shrunk to a point, and this space must be a three-dimensional sphere.

Later, this conjecture was extended to more than three-dimensional space, known as the "high-dimensional Poincaré conjecture".

6. Riemann's conjecture: "Not only are there infinitely many prime numbers, but these infinitely many prime numbers appear in a subtle and precise pattern ." ( Unresolved, related to prime numbers )

→ Riemann function

Referenced Articles (Thanks to the authors of the following articles):

[1] Mathematics Culture Appreciation Study Notes_violet forever's blog-CSDN blog

[2] [Study Notes] MOOC Mathematical Culture Appreciation Notes_Feeding Salt Blog-CSDN Blog

[3] Mathematics Culture Appreciation Final Notes_NP_hard's Blog-CSDN Blog

Mathematics Culture Appreciation MOOC Notes 【2023】

01 The soul of mathematics

1. Object and content of mathematics